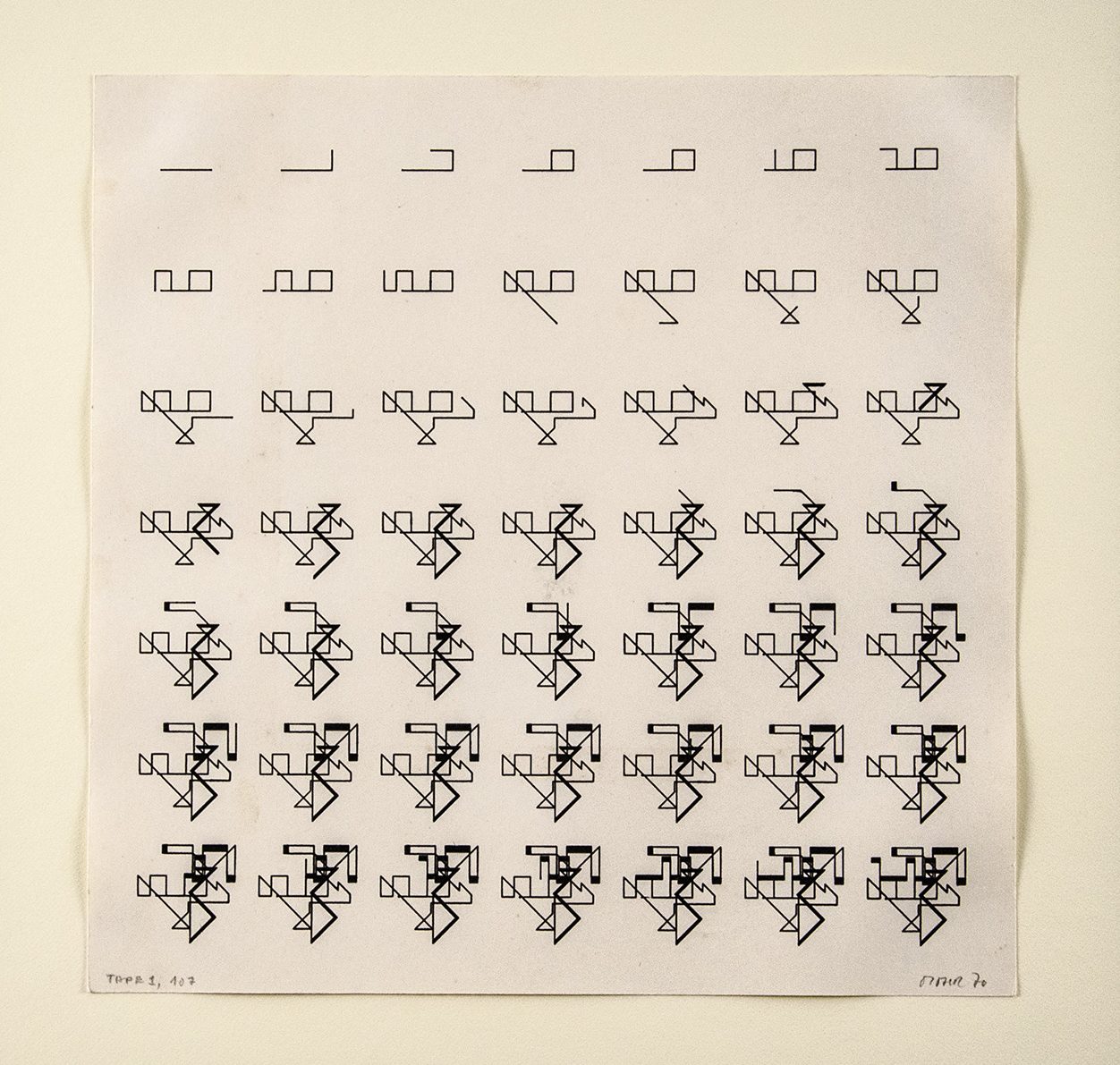
P-026 Inversion Logique (Logical Inversion)

Basic Information

Title: P-026 Inversion Logique (Logical Inversion)
Artist(s): Manfred Mohr
Date Created: 1970
Unframed Dimensions: 9 x 9 in.
Medium:
Inventory ID: Mohr-1970-05

Description

signed and dated lower right in graphite
“Tape 1, 107” written in graphite lower left
titled lower left on the reverse of the paper in graphite

hardware: CDC7600
software: Program 26 with FORTRAN
output machine: Benson Plotter

information bout the algorithm provided by the artist:
The elements are horizontal, vertical, 45 degree lines, square waves, zigzags, and have probabilities for line widths and lengths.
For each position in a matrix (left to right, top to bottom), the algorithm randomly chooses a new element from the alphabet to add to the existing elements.
In P-26, when the middle position is reached, an element is subtracted from the composite sign in the order in which it was added. Thus the last position in the grid shows the last element added.
In P-28, elements are continually added until the last grid position.

 

More Artworks By Manfred Mohr

XY-Plane P-308-3088A Triptych

Created in 1985 by Manfred Mohr

each drawing is signed lower right in graphite each drawing is titled lower left in graphite each drawing is titled and dated on the reverse of the frame program created in 1980 drawing completed in 1985 22.5 x 22.5″ each 22.5 x 67.5″ overall


P-181D Triptych

Created in 1979 by Manfred Mohr

each drawing is signed and dated lower right in graphite each drawing is titled lower left in graphite program created in 1975 drawing completed in 1979 19.25 x 19.25″ each 19.25 x 57.75″ overall


P-021+

Created in 1970 by Manfred Mohr

signed and dated lower right in graphite titled lower left in graphite artist’s name, title, date, measurements, and medium printed on artist’s label attached to the back of the frame